From behavior to bio-inspiration: Aerial reorientation and multi-plane stability in kangaroo rats, computational models, and robots
Abstract
Tails play essential roles in functions related to locomotor stability and maneuverability among terrestrial and arboreal animals. In kangaroo rats, bipedal hopping rodents, tails are used as effective inertial appendages for stability in hopping, but also facilitate stability and maneuverability during predator escape leaps. The complexity of tail functionality shows great potential for bio-inspiration and robotic device design, as maneuvering is accomplished by a long and light-weight inertial appendage. To understand (i) further understand the mechanics of how kangaroo rats use their tails during aerial maneuvers, and to (ii) explore if we can achieve this behavior with a simplified tail-like appendage (i.e., template), we combined quantified animal observations, computational simulations, and experiments with a two degrees of freedom (2-DoF) tailed robot. We used video data from free-ranging kangaroo rats escaping from a simulated predator and analyzed body and tail motion for the airborne phase. To explain tail contributions to body orientation (i.e., spatial reorientation), we built a mid-air kangaroo rat computational model and demonstrate that three-dimensional body orientation of the model can be controlled by a simplified 2-DoF tail with a nonlinear control strategy. Resulting simulated trajectories show movement patterns similar to those observed in kangaroo rats. Our robot experiments show that a lightweight tail can generate a large yaw displacement and stabilize pitch and roll angles to zero, simultaneously. Our work contributes to better understanding of the form-function relationship of the kangaroo rat tail and lays out an important foundation for bio-inspiration in robotic devices that have lightweight tail-like appendages for mid-air maneuvering.
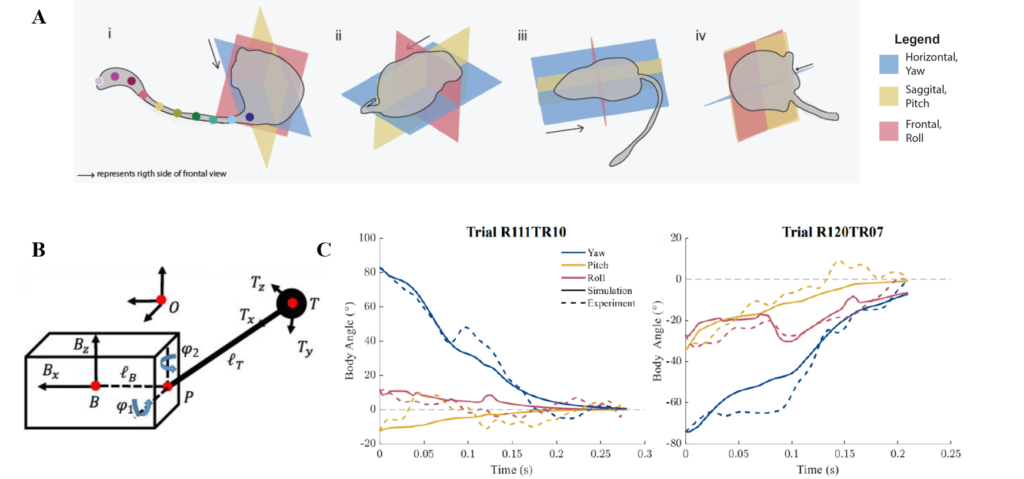
(A) Kangaroo rat aerial maneuver tracking in three planes. (B) Schematic representation of the two-segment control model. (C) Kangaroo rat body reorientation data and computation simulation results from two representative trials. The animal data, obtained from 3D kinematics, are indicated by dashed lines, while simulation results are shown in solid lines. We showed here that the computational body angle change had the same tendencies as the animal’s body, suggesting a well-fitting model. This implies that a simplified robot with a nonlinear control model, as we presented here, can mimic the kangaroo rat’s aerial reorientation and multi-plane stability during an escape response.
